Simultaneous Observations of Mesospheric Gravity Waves
and Sprites Generated by a Midwestern Thunderstorm
Sentman, D.D.1, E.M. Wescott1, R.H. Picard2, J.R. Winick2, H.C. Stenbaek-Nielsen1,
E.M. Dewan2, D.R. Moudry1, F.S. São Sabbas1, and M.J. Heavner3
1. Geophysical Institute, University of Alaska, Fairbanks, AK 99775, USA
2. Air Force Research Laboratory, Space Vehicles Directorate, Hanscom AFB, MA, USA
3. Los Alamos National Laboratory, NIS-1, Los Alamos, NM, USA
Abstract
The present report investigates using simultaneously observations of coincident gravity waves and sprites to determine the sprite-associated thermal energy deposition in the mesosphere. The University of Alaska operated a variety of optical imagers and photometers at two ground sites in support of the NASA Sprites99 balloon campaign. One site was atop a U.S. Forest Service lookout tower on Bear Mt. in the Black Hills, in western South Dakota. On the night of 18 August 1999 we obtained from this site simultaneous images of sprites and OH airglow modulated by gravity waves emanating from the same underlying thunderstorm. Using 25 second exposures with a bare CCD camera equipped with a red filter, we were able to coincidentally record both short duration (<10 ms) but bright (> 3 MR) N2 1PG red emissions from sprites and the much weaker (~1 kR), but persistent, OH Meinel nightglow emissions. A time lapse movie created from images obtained looking southeast from Bear Mt. toward a very active sprite-producing thunderstorm over Nebraska revealed short period concentric wave structures emanating radially outward from a central excitation region directly above the storm. During the initial stages of the storm outwardly expanding waves possessed a period of ![]() min and wavelength
min and wavelength ![]() km. Over a 1 hr interval the waves gradually changed to longer period
km. Over a 1 hr interval the waves gradually changed to longer period ![]() min and shorter wavelength
min and shorter wavelength ![]() km. Over the full 2 hr observation period, about two dozen bright sprites generated by the same underlying thunderstorm were recorded near the center of the outwardly radiating gravity wave pattern. No distinctive OH brightness signatures uniquely associated with the sprites were detected at the level of 2% of the ambient background brightness, establishing an upper limit of approximately
km. Over the full 2 hr observation period, about two dozen bright sprites generated by the same underlying thunderstorm were recorded near the center of the outwardly radiating gravity wave pattern. No distinctive OH brightness signatures uniquely associated with the sprites were detected at the level of 2% of the ambient background brightness, establishing an upper limit of approximately ![]() 0.5 K neutral temperature perturbation over the volume of the sprites. The total optical energy of the associated sprites is estimated to be 1.7-2.5 MJ, with a corresponding total energy of 170-2500 MJ.
0.5 K neutral temperature perturbation over the volume of the sprites. The total optical energy of the associated sprites is estimated to be 1.7-2.5 MJ, with a corresponding total energy of 170-2500 MJ.
1. Introduction
Sprites are transient (several to 10ās of ms) optical emissions generated within the mesosphere (50-90 km) by large lightning discharges [e.g., Sentman and Wescott, 1993; Lyons, 1994; cf. Sentman, 1998]. Several mechanisms have been proposed for sprite production [see review by Rowland, 1998], all based on impact excitation of ambient molecular species by electrons accelerated in transient mesospheric electric fields following a lightning discharge in the underlying thunderstorm. Imaging and photometric measurements reveal a continuous range of sprite brightnesses. These range from a few tens kR for small events to several MR for the brightest forms [Moudry et al., 2001], corresponding to total optical energies of a few tens kJ to several MJ, respectively, for storms of area 300-400 km2 (see Section 5.4.2 below for a review of the method for converting optical brightness to energy). Spectroscopic studies have shown the optical emissions of sprites are predominantly from the red ![]() 1PG bands of neutral molecular nitrogen [Hampton et al., 1996; Mende et al, 1995; Heavner, 2000]. Weak red
1PG bands of neutral molecular nitrogen [Hampton et al., 1996; Mende et al, 1995; Heavner, 2000]. Weak red ![]() Meinel emissions have been reported [Bucsela et al., 1998; Morrill et al., 1998] as well as transient
Meinel emissions have been reported [Bucsela et al., 1998; Morrill et al., 1998] as well as transient ![]() 1NG blue signatures of ionization [Armstrong et al., 1998; Suszcynsky et al., 1998; Takahashi et al., 1998; Armstrong et al., 2000].
1NG blue signatures of ionization [Armstrong et al., 1998; Suszcynsky et al., 1998; Takahashi et al., 1998; Armstrong et al., 2000].
An outstanding question regarding possible consequences of sprite occurrence within the middle and upper atmosphere is the total energy deposited in this region in comparison with other external energy inputs, such as by solar EUV or auroral particle precipitation from above, or by gravity waves from below. The N2 1PG bands observed in sprites correspond to the electric dipole transitions ![]() and constitute an optical marker that a substantial perturbation to the underlying medium has been created by the accompanying lightning discharge. The N2 1PG emissions occur because the spontaneous emission lifetime between the upper B and lower A excited electronic states is shorter than the collisional quenching time. They are visible at the ground because the atmosphere is relatively transparent at this wavelength. Electron impact excitation and ionization of O2 are also believed to occur [Pasko et al., 1997], but collisional quenching suppresses optical emissions from these species. Other excited states in the medium are presumably also created by electron impact, but are efficiently quenched by neutral collisions.
and constitute an optical marker that a substantial perturbation to the underlying medium has been created by the accompanying lightning discharge. The N2 1PG emissions occur because the spontaneous emission lifetime between the upper B and lower A excited electronic states is shorter than the collisional quenching time. They are visible at the ground because the atmosphere is relatively transparent at this wavelength. Electron impact excitation and ionization of O2 are also believed to occur [Pasko et al., 1997], but collisional quenching suppresses optical emissions from these species. Other excited states in the medium are presumably also created by electron impact, but are efficiently quenched by neutral collisions.
Besides exciting the electronic states, the characteristic electron energy of a few eV leading to the N2 1PG emissions should also result in substantial vibrational and rotational excitation of the ambient species, both within the observed transition states of N2 [Cartwright, 1978; Green et al., 1996] and within a larger group of vibrational and rotational states of both N2 and O2. Thus, while the N2 1PG emissions constitute the bulk of the optical energy observed, they are likely to represent only a small fraction of the total energy deposited in the mesosphere at the location of a sprite.
Sprites typically extend to altitudes of 85-90 km [Sentman et al., 1995], and thus penetrate into the airglow regions maintained in the mesosphere by a balance of various chemical and transport processes. The visible volume of large sprites often exceeds 104 km3 for large events [Sentman and Wescott., 1993]. Their duration is short (few ms), so thermal energy deposited in the neutral atmosphere within a sprite would be expected to produce an impulsive pressure pulse that propagates laterally outward as an acoustic wave or gravity wave. The amount of neutral heating has been previously estimated to be small (~0.3 to 3K; Pasko et al., 1997) for sprites of brightness ~1 MR, but this estimate has model-dependent uncertainties and has not been observationally confirmed. The motivation for the present observations was to search for a temperature perturbation signature associated with sprites by way of their associated effects on the nightglow emissions [Sentman et al., 1999]. Detection of such sprite correlated nightglow pulses would provide a method for indirectly determining the underlying thermal energy deposition associated with the sprites.
2. OH Chemistry Background
Of particular interest to this study is the OH nightglow layer located at ~85 km. The production of vibrationally excited hydroxyl, or OH(v), in the nighttime mesosphere is due to a series of chemiluminescent reactions between odd oxygen and odd hydrogen species [Makhlouf et al., 1995],

\* MERGEFORMAT ()1
where T is the ambient neutral temperature in degrees K, and k1...k5 are rate coefficients in cm3/s for two-body reactions and cm6/s for three-body reactions. Near the mesopause the neutral temperature is T ~ 170K. The vibrationally excited states can be quenched by collision with major species or chemically by the OH(v) + O reaction above, but the major loss over most of the region of interest is by radiation,
![]() (1ā)
(1ā)
where ![]() is the Einstein coefficient for spontaneous emission, v = 1,·,9, and
is the Einstein coefficient for spontaneous emission, v = 1,·,9, and ![]() . The observed radiance
. The observed radiance ![]() from the vibrational level v to level
from the vibrational level v to level ![]() transition is then the integral of the volume emission rate
transition is then the integral of the volume emission rate ![]() [OH(I)] along the line-of-sight, where [OH(v)] is the density of species OH in vibrational state v. The nighttime airglow, or nightglow, generated by these reactions produces brightest emissions (100s kR) from the
[OH(I)] along the line-of-sight, where [OH(v)] is the density of species OH in vibrational state v. The nighttime airglow, or nightglow, generated by these reactions produces brightest emissions (100s kR) from the ![]() first-overtone Meinel sequences at wavelengths 1.4-2.2 mm, with the total intensity of all emission sequences exceeding several MR (Chamberlain, 1961).
first-overtone Meinel sequences at wavelengths 1.4-2.2 mm, with the total intensity of all emission sequences exceeding several MR (Chamberlain, 1961).
Gravity waves produced by convective core motions of vigorous thunderstorms propagate into the high atmosphere and constitute a major source of mechanical energy and momentum into this region from below [Swenson and Liu, 1998]. Among other effects, these thunderstorm-generated gravity waves can modulate the optical emissions in the nightglow layer that are detectable from the ground [Taylor and Hapgood, 1988] and from orbiting imaging platforms [Dewan et al.,1998]. The emission intensity of the OH nightglow is sensitively dependent on small wave-induced temperature fluctuations [Makhlouf et al., 1995] and readily responds to thunderstorm-generated gravity wave pressure fluctuations to produce brightness variations such as reported by Taylor et al. [1995].
To determine the response of the OH nightglow emissions to a gravity wave, the coupled set of equations (1) and (2) is perturbed by a small temperature amplitude ![]() and the linear response of system determined. Because the rate coefficients in the reactions above possess different temperature dependencies, the equilibrium density of OH is also temperature dependent. The differential response of the OH Meinel emission intensities is proportional to the equilibrium OH density and may be expressed in terms of the differential temperature perturbation as
and the linear response of system determined. Because the rate coefficients in the reactions above possess different temperature dependencies, the equilibrium density of OH is also temperature dependent. The differential response of the OH Meinel emission intensities is proportional to the equilibrium OH density and may be expressed in terms of the differential temperature perturbation as
![]() , (2)
, (2)
where ![]() is a factor that depends on wave frequency, or equivalently, phase speed. For mesosphere conditions the factor
is a factor that depends on wave frequency, or equivalently, phase speed. For mesosphere conditions the factor ![]() ranges from
ranges from ![]() for
for ![]() =40 m/s to
=40 m/s to ![]() for
for ![]() = 140 m/s. Hence, a small temperature change produces a correlated OH intensity response amplified by a factor of 5-10. To scale optical emission variations to the underlying temperature effects requires knowledge of the adjustment time scale for the process, as given in the
= 140 m/s. Hence, a small temperature change produces a correlated OH intensity response amplified by a factor of 5-10. To scale optical emission variations to the underlying temperature effects requires knowledge of the adjustment time scale for the process, as given in the ![]() parameter above. OH emissions modulated by gravity waves provide the necessary information in their measurable phase speeds to determine this parameter.
parameter above. OH emissions modulated by gravity waves provide the necessary information in their measurable phase speeds to determine this parameter.
In the present study we searched for transient, in-situ heating effects at the mesopause produced by sprites through their effects on the modulation of the nocturnal OH airglow emissions, similar to the effects produced by gravity wave modulation of the OH emissions. While making the measurements of sprites above a very vigorous thunderstorm we unexpectedly captured a well defined example of an outwardly expanding, gravity wave driven concentric ripple pattern in OH emissions driven by the thunderstorm, similar to effects first reported by Taylor and Hapgood [1988], and further described by Dewan et al. [1998]. The dispersion relation extracted from these narrow band waves provided a key parameter for characterizing the perturbation response of OH needed to evaluate the sprite heating parameters.
3. Experiment
Observations were performed during August, 1999 as part of the NASA Sprites99 campaign [Bering et al., 1999] at a U.S. Forest Service fire observation tower on Bear Mt. (latitude 43.88N, longitude 103.75W, altitude 2150 m), located in the Black Hills near the city of Custer, South Dakota. The observing camera was a Photometrics SenSys Model 400 768 x 512 pixel x 12 bit unintensified CCD system equipped with a Kinoptic 5.7mm f/1.8 TEGEA wide angle lens. The CCD-lens combination provided a 60H x 40V degree field of view. We used a Schott RG-715 715-nm red filter to pass a selected band of wavelengths in the NIR region of the spectrum. When combined with the camera response, the camera-filter combination (NIR CCD) possessed an overall optical pass band of 715-920 nm FWHM. Figure 1 shows the respective response functions of the camera-filter system.
The OH nightglow emissions we observed are not the brightest in absolute terms among the several types of nocturnal skyglow. As noted above, the brightest emissions occur in the medium-infrared (MIR) ![]() first-overtone Meinel sequences 1.4-2.2 mm. However, the OH intensities are significant (several tens kR) in the shorter wavelength 700-900 nm near-infrared (NIR)
first-overtone Meinel sequences 1.4-2.2 mm. However, the OH intensities are significant (several tens kR) in the shorter wavelength 700-900 nm near-infrared (NIR) ![]() Meinel sequences [Yee et al., 1991] and are the emissions reported here. The CCD cooling requirements for detection of the NIR emissions are much less stringent than for MIR, and make it possible to use standard CCD technology operating near the upper limits of the camera wavelength sensitivity. Thus, one may use bare (unintensified) CCD technology for sufficiently long exposure times (tens of seconds) [e.g., Taylor and Hill, 1991; Taylor et al., 1991] with a relatively modest (T~ 0 C) amount of cooling.
Meinel sequences [Yee et al., 1991] and are the emissions reported here. The CCD cooling requirements for detection of the NIR emissions are much less stringent than for MIR, and make it possible to use standard CCD technology operating near the upper limits of the camera wavelength sensitivity. Thus, one may use bare (unintensified) CCD technology for sufficiently long exposure times (tens of seconds) [e.g., Taylor and Hill, 1991; Taylor et al., 1991] with a relatively modest (T~ 0 C) amount of cooling.
Table 1 lists the principal OH Meinel bands and their locations within the overall instrument pass band 700-900 nm. The pass band included the ![]()
![]() (740-780 nm) and
(740-780 nm) and ![]() (850-900 nm) sequences, so the system was also sensitive to the sprite 1PG emissions. Hence, we were able to image both OH nightglow emissions and sprites simultaneously.
(850-900 nm) sequences, so the system was also sensitive to the sprite 1PG emissions. Hence, we were able to image both OH nightglow emissions and sprites simultaneously.
The operational mode used in the present experiment involved performing 25 sec exposures followed by a 5 sec period to transfer the digital images from the camera to hard disk, yielding an effective image frame rate of 1/30 fps. The camera was connected via a PCI interface card to a desktop computer running the Microsoft Windows 98 operating system (subsequently updated to Windows 2000). Image acquisition and readout were controlled with a custom script running within the main Photometrics camera control program. The computer system timer, updated continuously over the Internet to maintain 100 ms accuracy absolute, was used to synchronize image acquisition. The resultant set of images was processed off line using a Matlab script for histogram equalization, and combined into a digital video clip using an ActiveX Automation script running under Corel PhotoPaint. A separate intensified CCD (ICCD) television system was also operated during the campaign to obtain detailed images of many of the brighter sprites that also registered in the NIR images.
4. Observations
On the night of 18 August 1999 over a two hr interval we obtained very clear images of both gravity wave modulated OH emissions and sprites associated with a very intense thunderstorm over Nebraska. The thunderstorm is shown in GOES infrared imagery with overlaid lightning strike information in Figure 2. Over the 3 hr period 0400-0700 UT, the center of storm convection and lightning activity moved approximately 200 km from south-central Nebraska to eastern Nebraska/Iowa at an average speed of about 60-70 km/hr (17-19 m/s). This storm track was roughly orthogonal to the observing line of sight, which approximately preserved the distance to the storm during the observing interval. Observing conditions were close to ideal, with a clear sky above the observing site permitting visual access to OH nightglow and sprite activity in the mesosphere above the thunderstorm.
Figure 3 shows one of the NIR CCD images obtained during the observation interval. In the foreground near the bottom of the large image are seen the lights of the city of Custer, SD, and to the left the display lights of the Crazy Horse Monument. Left lower center there is a large sprite, shown in high resolution as a cutout from the ICCD TV camera. The stars are clearly visible at the top of the figure. The hazy elliptical features in the lower central portion of the figure labeled as "Concentric Expanding Gravity Wave Ripples" are the gravity wave signatures we seek. When the 30 sec images are viewed as a video sequence, these elliptical features exhibit a distinctive outward propagation pattern originating from a well defined center.
The gravity wave structure shown in Figure 3 may be mapped onto a flat surface to determine the relationship of the various features in the figure to the underlying thunderstorm. We projected each pixel in the image onto the 85 km tangent plane assuming the analysis strip lay in the tangent plane. Figure 4 shows the resultant map of Figure 3 laid over the corresponding GOES weather map of the Nebraska storm. This projection clearly associates the gravity wave modulation structures with the underlying thunderstorm.
5. Analysis
5.1. Identification of OH Meinel Emissions
The video sequence clearly shows circular waves radiating outward from a region centered over the thunderstorm in eastern Nebraska. We interpret these propagating waves as being mesospheric nightglow emissions modulated by gravity waves generated by the underlying thunderstorm. There are several nightglow layers in the mesosphere that are candidate sources for the observed emissions. Only two fall within our pass band. The 700-900 nm ![]() Meinel sequences indicated in Figure 1 emit from a thin layer centered at an altitude of ~85 km. The bright (0ö0, 1) components of the O2 Atmospheric bands arising from
Meinel sequences indicated in Figure 1 emit from a thin layer centered at an altitude of ~85 km. The bright (0ö0, 1) components of the O2 Atmospheric bands arising from ![]() (761.9, 859.8 nm) also fall within our pass band, but the atmosphere is optically thick for these emission so they are not observed at the ground. The remaining two nightglow sources, the O2
(761.9, 859.8 nm) also fall within our pass band, but the atmosphere is optically thick for these emission so they are not observed at the ground. The remaining two nightglow sources, the O2![]() Infrared Atmospheric bands and the sodium Na I (2P
Infrared Atmospheric bands and the sodium Na I (2P![]() 1S
1S![]() ) + h (589 nm) D-line emissions, fall outside the instrument pass band. We conclude that the observed emissions are predominantly from the OH Meinel bands.
) + h (589 nm) D-line emissions, fall outside the instrument pass band. We conclude that the observed emissions are predominantly from the OH Meinel bands.
5.2 Characterization of Gravity Wave Parameters
5.2.1 Analysis Method
To determine the phase speed and wavelength of the outwardly expanding ripples, we established a horizontal analysis strip across the center of the ripple pattern in the image, indicated by a set of horizontal dashed lines passing through the center of the circular ripple patterns in Figure 3. For each column the intensity was summed across 4 rows of the strip to produce a horizontal emission profile. By assuming the emissions originate at an altitude of 85 km corresponding to the OH layer, this could be converted to the corresponding emission profile as a function of horizontal distance in km. One such profile was computed for each 30 second image during the observing interval.
Figure 5 shows the results of stacking horizontal emission profiles from successive images into a color-coded position-time plot, where the horizontal scale is the position in km from the left edge of the image at the position of the dashed lines in Figure 3. Time runs vertically from bottom to top beginning at 0400 UT. The outward propagation of the waves from the central region of the figure with increasing time is clearly discernible in this plot. The short upward streaks in the plot are stars as they pass through the (fixed) analysis strip. Sprites intersecting the analysis strip produce signatures of short horizontal strips of width equal the width of the sprite. However, not every sprite that was captured in the images intersected the analysis strip, so the number of sprite signatures seen here undercounts the actual number observed of approximately 20.
5.2.3 Wave Period and Horizontal Wavelength
The wave period and horizontal wavelength may be read directly from Figure 5, and are approximately ![]() 8-11 min and
8-11 min and ![]() 40 km, respectively, at 1 hr into the plot. The corresponding phase speed is 60-70 m/s. This period and wavelength differ from the periods of 5-13 min and wavelengths of 14-25 km previously reported [Taylor and Hill, 1991], but the phase speed is comparable. In Section 5.2.5 below we analyze the time evolution of the propagation parameters that is evident in Figure 5 between the beginning and the end of the analysis interval.
40 km, respectively, at 1 hr into the plot. The corresponding phase speed is 60-70 m/s. This period and wavelength differ from the periods of 5-13 min and wavelengths of 14-25 km previously reported [Taylor and Hill, 1991], but the phase speed is comparable. In Section 5.2.5 below we analyze the time evolution of the propagation parameters that is evident in Figure 5 between the beginning and the end of the analysis interval.
5.2.4 Vertical Wavelength
The horizontal component cph of phase velocity cp is measured to be cph » 60 m/s and horizontal wavelength l h » 40 km. These results assume emission is from an altitude of 85 km. The resulting period t is 670 sec, or 11 min, also visible in the plot.
From this information and the properties of gravity waves, one can infer other characteristics of the waves including the vertical wavelength l z. Gravity waves originating in the lower atmosphere propagate upward with a group velocity cg which is orthogonal to the phase velocity cp, or the wave vector k = (kh, kz) = (2p /l h, 2p /l z), where kh and kz are the horizontal and vertical wave numbers, and l h and l z are the corresponding wavelengths, respectively. Hence, cg is directed along the phase fronts, and the angle of elevation j of the group velocity is given by
![]() (3)
(3)
Small-scale waves (cpx << cs, the speed of sound) satisfy the dispersion relation
![]() , (4)
, (4)
where N is the Brunt-Vaisala frequency and w = 2p /t is the gravity wave frequency, from which it follows that
 . (5)
. (5)
Assuming a Brunt period t B = 2p /N » 5 min and using (3), expression (5) yields
![]() , (6)
, (6)
with the result that ![]() = 27o and
= 27o and ![]() = 20 km.
= 20 km.
Assuming the emissions originate in the OH Meinel bands, the vertical thickness H of the emission layer is roughly 8 km [cf. Makhlouf et al., 1995]. Hence, the phase of the wave changes very little in the z direction across the layer ![]() , and one would expect to have little "observational filtering" of the wave modulation by destructive interference or cancellation effects of emissions originating from different portions of the emitting layer. This is consistent with the observed gross spatial structure of the gravity wave emissions, where there is little variation in the modulation amplitude across the entire structure. By way of contrast, if the vertical wavelength
, and one would expect to have little "observational filtering" of the wave modulation by destructive interference or cancellation effects of emissions originating from different portions of the emitting layer. This is consistent with the observed gross spatial structure of the gravity wave emissions, where there is little variation in the modulation amplitude across the entire structure. By way of contrast, if the vertical wavelength ![]() is less than H, the portions of the pattern where the line-of-sight was aligned more closely with the wave fronts would be brighter [Alexander and Picard, 1999]. Such a thunderstorm-generated wave pattern with varying contrast can be seen in the MSX satellite observations of stratospheric gravity waves in 4.3-mm CO2 emission [Dewan et al., 1998]. Here, however, the effective thickness of the emitting layer is much greater (15-20 km) [Picard et al., 1998].
is less than H, the portions of the pattern where the line-of-sight was aligned more closely with the wave fronts would be brighter [Alexander and Picard, 1999]. Such a thunderstorm-generated wave pattern with varying contrast can be seen in the MSX satellite observations of stratospheric gravity waves in 4.3-mm CO2 emission [Dewan et al., 1998]. Here, however, the effective thickness of the emitting layer is much greater (15-20 km) [Picard et al., 1998].
5.2.5 Time Evolution
Over the observation interval the horizontal phase speed of the waves very clearly diminishes, as shown in Figure 5. At times near 70 min the slopes marked in this figure indicate a phase velocity cph of about 60 m/s, corresponding to a horizontal wavelength ![]() km. and a period
km. and a period ![]() = 11 min . However, at the bottom of this figure, near the beginning of the analysis interval at ~10 min or less, the slope is flatter, indicating a phase velocity of about 85 m/s and a horizontal wavelength of about 50 km. Using
= 11 min . However, at the bottom of this figure, near the beginning of the analysis interval at ~10 min or less, the slope is flatter, indicating a phase velocity of about 85 m/s and a horizontal wavelength of about 50 km. Using ![]() we obtain
we obtain ![]() 9.8 min; thus both wave frequency and wavelength were higher in the earlier part of the storm. Using equations (5) and (6) we can calculate
9.8 min; thus both wave frequency and wavelength were higher in the earlier part of the storm. Using equations (5) and (6) we can calculate ![]() from the values of
from the values of ![]() and
and ![]() . For the early time we find
. For the early time we find ![]() = 30 km. This is in contrast to the value
= 30 km. This is in contrast to the value ![]() = 20 km that is found for the later time.
= 20 km that is found for the later time.
In Table 2 we summarize these parameters and compare them with previous results reported by Taylor and Hapgood [1988] and results obtained from MSX observations [Dewan et al. [1998].
Wavelength parameters have been related to the physical properties of thunderstorms by Alexander et al. [1995] and Pandya and Alexander [1999]. The period t is the period of "mechanical oscillation" of the thunderstorm, i.e. the period of the oscillating convective updrafts and downdrafts within the storm that impinge upon the tropopause. The value of ![]() was shown by Salby and Garcia [1987] to be determined by the characteristic vertical dimension of ~10 km thickness of the thunderstormās thermal forcing layer in the troposphere. In fact the vertical extent of the storm should correspond to a half wavelength
was shown by Salby and Garcia [1987] to be determined by the characteristic vertical dimension of ~10 km thickness of the thunderstormās thermal forcing layer in the troposphere. In fact the vertical extent of the storm should correspond to a half wavelength ![]() , but in propagating from the troposphere to the stratosphere the wavelength shortens by about a factor of 2 (see Alexander et al. [1995]), so that
, but in propagating from the troposphere to the stratosphere the wavelength shortens by about a factor of 2 (see Alexander et al. [1995]), so that ![]() in the stratosphere and above is on the order of the vertical extent of the storm. On the basis of these considerations we see from Table 2 that the oscillation frequency and vertical extent of the storm both decrease in time during the life of the storm. The values of 20 km and 30 km inferred from the observations appear to be too large to represent actual storm extents, but the expected qualitative evolution of the thunderstorm parameters, consisting of slowing of the mechanical oscillation and shrinking of the stormās vertical dimension, seems to be firmly established by the present measurements.
in the stratosphere and above is on the order of the vertical extent of the storm. On the basis of these considerations we see from Table 2 that the oscillation frequency and vertical extent of the storm both decrease in time during the life of the storm. The values of 20 km and 30 km inferred from the observations appear to be too large to represent actual storm extents, but the expected qualitative evolution of the thunderstorm parameters, consisting of slowing of the mechanical oscillation and shrinking of the stormās vertical dimension, seems to be firmly established by the present measurements.
5.3 Other Wave Characteristics
From the wave propagation elevation angle j
, the ground range between the source and the region where the wave pattern is observed in the nightglow may be determined. If we assume rectilinear propagation without refraction from wind shears, for a thunderstorm source altitude of 15 km near the tropopause and an OH emission altitude of 85 km the ground range is 70 km/0.51 ![]() 140 km. As can be seen in Figure 5 this corresponds roughly to the radius of the observed wave pattern, so the characteristic horizontal scale size of the gravity wave effects is on the order of a few times the height of the nightglow layer above the thunderstorm source.
140 km. As can be seen in Figure 5 this corresponds roughly to the radius of the observed wave pattern, so the characteristic horizontal scale size of the gravity wave effects is on the order of a few times the height of the nightglow layer above the thunderstorm source.
The transit time from the source to the altitude where the effects are observed may be estimated to be approximately 70 km/cgz, where cgz is the vertical component of the group velocity of the gravity wave, given by cgz º ¶ w /¶ kz = (lz /t) cos2f, where w =2p /t is the wave frequency [Dewan et al., 1998]. Substituting the current values of the wave parameters, we calculate the vertical transit time from the thunderstorm to the mesopause OH layer to be approximately 40-50 min. Hence, in comparing thunderstorm characteristics and gravity wave parameters, the candidate storms which can act as sources should be determined by looking at GOES images 40-50 min before the wave observation.
Over the observation interval the underlying thunderstorm moved at a speed of approximately 15-20 m/s, roughly 0.2-0.3 the horizontal phase speed of the gravity waves. The region of the nightglow layer lying directly above the thunderstorm source moves with the thunderstorm, which from Figure 3 is seen to be predominantly from right to left as observed from Bear Mt. looking southeastward. This movement of the thunderstorm gravity source is reflected in the nightglow modulations, which can be seen in Figure 5 as a right-to-left drift in the brightest portion of the emissions as time progresses from bottom to top.
5.4 OH Brightness Perturbations and Associated Temperature Perturbations
5.4.1 Gravity Waves
Absolute intensity calibrations were not available for the Photometrics camera, but the intensities of the OH emissions may be estimated from the brightness of the sprites simultaneously recorded with the broad band, calibrated high speed imager operated during the Sprites99 campaign [Stenbaek-Nielsen et al., 2000]. Sprites typically persist for a few (~3-10 ms) and saturate the ICCD at 3 MR. The images obtained with the Photometrics NIR CCD register the apparent nightglow brightness to be approximately a third that of the sprites. By taking into account the sprite duration (10 ms) and the image integration time (30 sec), the brightness of the gravity wave modulated OH emissions is estimated to be (1/3)(10 ms/30 sec)(3 MR) ~ 300 R. This brightness appears to underestimate by about a factor of 3 previously reported OH brightness of 1-2 kR over a similar wavelength pass band reported by Yee et al. [1991] for observations made during the ALOHA-90 campaign. The discrepancy may arise from our use of the 3 MR saturation level of the cameras [Stenbaek-Nielsen et al., 2000]. If a more realistic intensity of 10 MR is used for the actual intensities of the sprites that saturated the camera, then the estimated OH intensities agree with the Yee et al. [1991] results. We shall henceforth use 1 kR for the mean brightness of the OH nightglow in our observations.
The optical modulation amplitude of the outwardly expanding waves was measured to be approximately 30% peak to peak compared to the background mean emission intensity. From Equation (2) and using values of the phase speed given in Table 2, we estimate ![]() . The resultant temperature perturbation associated with the concentrically expanding waves is ~4% of the ambient, or ~7K.
. The resultant temperature perturbation associated with the concentrically expanding waves is ~4% of the ambient, or ~7K.
We examined the observations carefully for the occurrence of small, radially expanding ripples originating from the point where sprites penetrated the OH nightglow layer. In Figure 5 they would be expected to appear as upward-slanting "wings" originating from each sprite that fell within the analysis strip. No sprite signatures of this type were detected in the OH emissions at the level of 2% of the background at that location. Using an assumed OH brightness of ~1 kR this negative result permits us to establish an observational upper limit of ~20 R for the brightness perturbation in the OH produced by a large sprite at altitudes ~85 km. Using Equation with From equation (2) et seq. and using values of the phase speed given in Table 2, we estimate ![]() the corresponding brightness perturbation
the corresponding brightness perturbation ![]() translates to an upper limit for the corresponding neutral temperature perturbation
translates to an upper limit for the corresponding neutral temperature perturbation ![]() 0.003, or
0.003, or ![]() 0.5 K at the altitude of the mesopause. The upper limit of
0.5 K at the altitude of the mesopause. The upper limit of ![]() 0.5 K is consistent with the thermal heating of
0.5 K is consistent with the thermal heating of ![]() 0.3-3 K estimated by Pasko et al. [1997] for large sprites of similar (> 1 MR) brightness.
0.3-3 K estimated by Pasko et al. [1997] for large sprites of similar (> 1 MR) brightness.
The upper limit ![]() 0.5 K for the temperature perturbation may be converted to an upper limit for the thermal energy deposited into the local medium by the sprite. If we assume that most of the energy associated with electron impact excitation is ultimately transferred by collisions with neutrals to thermal energy, then the total energy associated with this process may be estimated as
0.5 K for the temperature perturbation may be converted to an upper limit for the thermal energy deposited into the local medium by the sprite. If we assume that most of the energy associated with electron impact excitation is ultimately transferred by collisions with neutrals to thermal energy, then the total energy associated with this process may be estimated as ![]() , where Nn is the number density of neutrals,
, where Nn is the number density of neutrals, ![]() J/K is Boltzmannās constant, and V is the effective volume of the portion of the sprite intersecting the OH layer. This volume is estimated using the vertical thickness ~7 km of the OH emission layer and a horizontal cross section of 108 m2 of the sprite where it penetrates the OH layer, yielding V~7 x 1011 m3. With neutral density Nn=2 x 1020/m3 at an altitude of 85 km and DT<0.5 K, we arrive at an upper limit DE<1 GJ for the associated total thermal energy deposited in the OH layer.
J/K is Boltzmannās constant, and V is the effective volume of the portion of the sprite intersecting the OH layer. This volume is estimated using the vertical thickness ~7 km of the OH emission layer and a horizontal cross section of 108 m2 of the sprite where it penetrates the OH layer, yielding V~7 x 1011 m3. With neutral density Nn=2 x 1020/m3 at an altitude of 85 km and DT<0.5 K, we arrive at an upper limit DE<1 GJ for the associated total thermal energy deposited in the OH layer.
The energy deposition upper limit DE<1 GJ in the nightglow layer inferred from the absence of OH signatures may be compared to the total energy deposition at all altitudes within the mesosphere from the same events. Heavner et al. [2000] have previously estimated the total energy deposited in the mesosphere by bright sprites to be on the order of 1 GJ. The total energy may be estimated using the definition of brightness given by Chamberlain [1961, Appendix II]. Apparent brightness B(R), in Rayleighs, as seen by the observer is related to volume emissivity F(r), in photons/cm3 sec, in the source region by ![]() , where the integral is taken along the line of sight through the source. The source brightness is assumed to fill the detector aperture, which accounts for the absence of a 1/r2 factor in the integrand. If we approximate the emission region as a homogeneous, optically thin slab of thickness d along the line of sight, then
, where the integral is taken along the line of sight through the source. The source brightness is assumed to fill the detector aperture, which accounts for the absence of a 1/r2 factor in the integrand. If we approximate the emission region as a homogeneous, optically thin slab of thickness d along the line of sight, then ![]() , giving the volume emissivity
, giving the volume emissivity ![]() . For a brightness of 3 MR (e.g., Stenbaek-Nielsen et al., 2000) from a source of uniform emissivity along a slant path of length d=10 km, we obtain F(r) = 3 x 108 photons/cm3 sec = 3 x 1014 photons/m3 sec. The total optical energy associated with the observed brightness is approximately E = F(r) (hc/l) V Dt, where h is Planckās constant, c the speed of light, l the photon wavelength, and Dt the duration of the emissions. Using F(r) =3 x 1014 photons/m3 sec from above and assuming V = (10 km)3 = 1012 m3 for the volume of optical emissions, a mean wavelength of 700 nm for N2 1PG, and a duration Dt = 10 ms, we obtain E = 8.5 x 105 J for the total optical energy emitted by the sprite.
. For a brightness of 3 MR (e.g., Stenbaek-Nielsen et al., 2000) from a source of uniform emissivity along a slant path of length d=10 km, we obtain F(r) = 3 x 108 photons/cm3 sec = 3 x 1014 photons/m3 sec. The total optical energy associated with the observed brightness is approximately E = F(r) (hc/l) V Dt, where h is Planckās constant, c the speed of light, l the photon wavelength, and Dt the duration of the emissions. Using F(r) =3 x 1014 photons/m3 sec from above and assuming V = (10 km)3 = 1012 m3 for the volume of optical emissions, a mean wavelength of 700 nm for N2 1PG, and a duration Dt = 10 ms, we obtain E = 8.5 x 105 J for the total optical energy emitted by the sprite.
Applying the factor ~100-1000 for the ratio of total to optical energy in a manner similar to Heavner et al. [2000] to account for energy residing in the non-emitting rotational and vibrational states, we obtain a total estimated energy of 85-850 MJ, comparable to or slightly smaller than the earlier estimates.
The estimated upper limit of 1 GJ for the thermal energy deposited in the OH layer, obtained in Section 5.4.1 above, exceeds the total energy 85-850 MJ by a factor 1.2-12, so we conclude that either (a) the actual energy deposited in the OH layer is considerably smaller than the estimated upper limit, or (b) the estimate of the total energy for the sprite as a whole is considerably larger than the 85-850 MJ computed using a brightness of 3 MR, or possibly some combination of the two. For the second possibility (b), we note that the 3 MR brightness used in this calculation corresponds to the saturation level of the camera used to record the events reported by Stenbaek-Nielsen et al. [2000] and, as noted above in the assumptions used to estimate the OH brightness, may underestimate the true brightness of many of the observed events. The total optical energy E = 850 kJ computed here, therefore, may actually be larger by a factor of 2-3, raising the computed optical energy to 1.7-2.5 MJ and the total energy of the events to 170-2500 MJ. Additional observations that permit actual detection of OH intensity perturbations and a more accurate determination of the energy invested in molecular rotational and vibrational states are needed to resolve this ambiguity.
6. Conclusions
We have performed a simplified analysis on the gravity wave structures observed with the sprites to determine the basic wavelength and period parameters, and to extract the corresponding temperature perturbations implied by these parameters. The principal results of the present investigation may be summarized as follow:
The negative results obtained in the search for sprite-induced perturbations of OH emissions provide an important check on the theoretical predictions of thermal heating of the mesospheric neutral atmosphere. The upper limits for the temperature perturbation obtained here are broadly consistent with models, and suggest that detection of the effects on OH may be achievable using improved observation techniques.
While the principal thrust of the present work was to use gravity wave signatures in airglow as a tool to extract the energy deposition of sprites in the mesosphere, the image data set provides a valuable resource in its own right for studying the gravity waves associated with the storm of 18 August 1999. Additional analyses that are possible with this data include estimating the power in the waves and comparing it with what is available in the storm, considering the efficiency of the coupling to the atmosphere. This requires taking into account the wave-generation mechanisms. The three principal mechanisms by which storms can generate waves are [Joan Alexander, private communication]:
(a) The moving mountain: Shear flow over a dome of air associate with a convective cell can launch "mountain" waves upward.
(b) Transient pumping: This is the mechanical forcing effect on the atmosphere from the body force associated with the moving expanding air in the cell. There are two limits. If the forcing is impulsive, then it contains all frequencies and will tend to generate a broad spectrum of waves. According to the dispersion relation, the waves will be segregated in direction by their frequency, with short-period waves propagating more nearly vertically and longer period waves propagating in a more horizontal direction. On the other hand, there is evidence that quasi-sinusoidal oscillations of the convective air column occur in thunderstorms. Such oscillations would give rise to periodic forcing and generate quasi-sinusoidal waves. These waves would be launched at an angle determined by the period of the forcing and the wave dispersion relation. We have tacitly assumed this situation above in Section 5.2, as well as in Dewan et al. [1998].
(c) Transient heat source: This is the driving term in the thermal equation from the heat release in the storm. It tends to generate waves of given vertical wavelength l z rather than given frequency or period. The heated air column generates waves whose half-wavelength l z/2 is equal to the overall length of the column, as discussed in Section 5.2.5.
These mechanisms all operate simultaneously with varying relative contributions to the total energy input into the wave field. For the present set of observations of concentrically expanding waves emanating from a point above a thunderstorm, the original explanation offered by Taylor and Hapgood [1988] appears to hold. In that report, the occurrence of concentric circular waves in the OH airglow assumed that the thunderstorm was a quasi-monochromatic source of gravity waves, and evidence for this was cited in the work of Anderson [1960]. Most recently, Piani et al. [2001] performed three-dimensional simulations of gravity waves generated by a large connective thunderstorm and obtained results in striking agreement with observations of Taylor and Hapgood [1988], Dewan et al. [1998], and the present work. Thus, the cause of the observed concentric patterns appears to be well understood.
Acknowledgments
This research was partially supported by NASA Grants NAG5-5019 and NAG5-0131 to the University of Alaska. RHP, JRW, and EMD are grateful for the support of Kent Miller of the Air Force Office of Scientific Research, and RHP acknowledges useful discussions with Joan Alexander. We extend special thanks to the U.S. Forest Service in Custer, SD for permitting us to use the lookout tower at Bear Mountain. This work was originally presented at the 1999 Fall Meeting of the American Geophysical Union (Sentman et al., 2000). The video clip exhibiting the gravity waves and sprites discussed here is available on request from dsentman@gi.alaska.edu.
References
Alexander, M.J., and R.H. Picard, Evaluating the importance of convectively generated waves observed by the MSX satellite to middle atmosphere dynamics, EOS Trans. Am. Geophys. Union, 80, F207, 1999.
Anderson, C.E., Cumulus Dynamics, Pergamon Press, New York, 1960
Alexander, M.J., J.R. Holton, D.R. Durran, The gravity wave response above deep convection in a squall line simulation, J. Atmos. Science, 52, 2212-2226, 1995.
Armstrong, R.A., J.A. Shorter, M.J. Taylor, D.M. Suszcynsky, W.A. Lyons and L.S. Jeong, Photometric measurements in the Spritesā95 and ā96 campaigns of nitrogen second positive (399.8 nm) and first negative (4278 nm) emissions, J. Atmos. Solar-Terr. Phys., 60, 787-799, 1998.
Armstrong, R.A., D.M. Suszcynsky, W.A. Lyons and T.E. Nelson, Multi-color photometric measurements of ionization and energies in sprites, Geophys. Res. Lett. 27, 653-656, 2000.
Bering, E A, Benbrook, J R, Garrett, J A, Paredes, A, Wescott, E M, Sentman, D D, Stenbaek-Nielsen, H C, and Lyons, W A, "The 1999 Sprites Balloon Campaign," Eos Trans. AGU, 82 , Fall Meet. Suppl., Abstract A42E-01, 1999.
Bucsela, E, J Morrill, C Siefring, M Heavner, D Moudry, D Sentman, E Wescott, D Osborne, W Benesch, Estimating electron energies in sprites from 1NG/2PG intensity ratios, EOS Trans. Am Geophys. Union, 81, Fall Meeting Suppl., Abstract A42-D04, 1998.
Cartwright, D.C., Vibrational populations of the excited states of N2 under auroral conditions, J. Geophys. Res., 83, 517-531, 1978.
Chamberlain, J.W., Physics of the Aurora and Airglow, Academic Press, New York, 1961.
Dewan, E.M., R.H. Picard, R.R. O'Neil, R. R., H.A. Gardiner, J. Gibson, J.D. Mill, E. Richards, M. Kendra, and W.O. Gallery, MSX satellite observations of thunderstorm-generated gravity waves in midwave infrared images of the upper stratosphere, Geophys. Res. Lett. 25, 939-942, 1998.
Fukunishi, H., Y. Takahashi, M. Jufito, Y. Watanabe and K. Sakanoi, Fast imaging of elves and sprites using a framing/streak camera and a multi-anode array photometer, EOS Trans. Am. Geophys. Union, 77, F60, 1996.
Green, B.D., M.E. Fraser, W.T. Rawlins, L. Jeong, W.A.M. Blumberg, S.B. Mende, G.R. Swenson, D.L. Hampton, E.M. Wescott and D.D. Sentman, Molecular excitation in sprites, Geophys. Res. Lett., 23, 2161-2164, 1996.
Hampton, D.L., M.J. Heavner, E.M Wescott and D.D. Sentman, Optical spectral characteristics of sprites, Geophys. Res. Lett., 23, 89-92, 1996
Heavner, M.J., D.D. Sentman, D.R. Moudry, E.M. Wescott, C.L. Siefring, J.S. Morrill, and E.J. Bucsela, "Sprites, Blue Jets, and Elves: Optical Evidence of Energy Transport Across the Stratopause," in Atmospheric Science Across the Stratopause (D. Siskind, editor), American Geophysical Union, Washington D.C., 2000.
Lyons, W.A., Characteristics of luminous structures in the stratosphere above thunderstorms as imaged by low-light video, Geophys. Res. Lett., 21, 875-878, 1994.
Makhlouf, U.B., R.H. Picard, and J.R. Winick, Photochemical-dynamical modeling of the measured response of airglow to gravity waves, J. Geophys. Res., 100, 11,289-11,311, 1995.
Mende, S.B., R.L. Rairden, and G.R. Swenson, Sprite spectra: N2 1PG band identification., Geophys. Res. Lett., 20, 2633-2536, 1995.
Morrill, J.S., E.J. Bucsela, V.P. Pasko, S.L. Berg, M.J. Heavner, D.R. Moudry, W.M. Benesch, E.M. Wescott, and D.D. Sentman, Time resolve N2 triplet state vibrational populations and emissions associated with red sprites," J. Atmos. Solar-Terr. Phys., 60, 811-830, 1998.
Moudry, D., H. Stenbaek-Nielsen, D. Sentman, and E. Wescott, On sprite initiation, J. Atmos. Solar-Terr. Phys., (this issue), 2001.
Pandya, R., and M.J. Alexander, Linear stratospheric gravity waves above convective thermal forcing, J. Atmos. Science, 56, 2434-2446,1999.
Pasko, V.P., U.S. Inan, T.F. Bell, and Y.N. Taranenko, Sprites produced by quasi-electrostatic heating and ionization in the lower ionosphere, J. Geophys. Res., 102, 4529, 1997.
Pearse, R.W.B., and A.G. Gaydon, The Identification of Molecular Spectra, (4th edition), Wiley, New York, 1976.
Piani, C., D. Durran, M.J. Alexander, and J.R. Holton, A numerical study of three dimensional gravity waves triggered by deep tropical convection and their role in the dynamics of Q.B.O., J. Atmos. Sci., 57, 3689-36702, 2001.
Picard, R.H., R.R. O'Neil, H.A. Gardiner, J. Gibson, J.R. Winick, W.O. Gallery, A.T. Stair, Jr., P.P. Wintersteiner, E.R. Hegblom, and E. Richards, Remote sensing of discrete stratospheric gravity-wave structure at 4.3-mm from the MSX satellite, Geophys. Res. Lett., 25, 2809-2812, 1998.
Rowland, H.L., Theories and simulations of elves, sprites and blue jets, J. Atmos. Solar-Terr. Phys., 60, 831-844, 1998.
Salby, M.L., and R.R. Garcia, Transient response to localized episodic heating in the tropics. Part 1: Excitation and short-time near-field behavior, J. Atmos. Science, 44, 458-497, 1987.
Suszcynsky, D.M., R.A. Roussel-Dupré, W.A. Lyons, and R.A. Armstrong, Blue light imagery and photometry of sprites, J. Atmos. Solar-Terr. Phys., 60, 801, 1998.
Sentman, D.D., and E.M. Wescott, Video observations of upper atmospheric optical flashes recorded from an aircraft, Geophys. Res. Lett., 20, 2857-2860, 1993.
Sentman, D.D., E.M. Wescott, D.L. Osborne, D.L. Hampton and M.J Heavner, Preliminary results from the Sprites94 aircraft campaign: 1. Red Sprites, Geophys. Res. Lett., 22, 1205-1208, 1995.
Sentman, D.D. (Editor), Effects of Thunderstorm Activity on the Upper Atmosphere and Ionosphere, Special Issue of J. Atmos. Solar-Terr. Phys., 60, ( Nos. 7-9), 1998.
Sentman
, D.D., E.M. Wescott, H.C. Stenbaek-Nielsen, M.J. Heavner, D.R. Moudry, and F.T. São Sabbas, Observations of gravity waves associated with a sprite-producing storm, Eos Trans. AGU, 82 (48), Fall Meet. Suppl., Abstract A12B-06, 2000.Stenbaek-Nielsen, H.C., D.R. Moudry, E.M. Wescott, D.D. Sentman and F.T. São Sabbas, Sprites and possible mesospheric effects, Geophys. Res. Lett., 27, 3829-3832, 2000.
Swenson, B.R., and A.Z. Liu, A model for calculating acoustic gravity wave energy and momentum flux in the mesosphere from OH airglow, Geophys. Res. Lett., 25, 477, 1998.
Takahashi, U., Y. Watanabe, A. Uchida, M. Sera, M. Sato and H. Fukunishi, Energy distributions of electrons exciting sprites and elves inferred from the Fast Array Photometer observations, EOS Trans. Am. Geophys. Union, 79, F175, 1998.
Taylor, M.J., and M.A. Hapgood, Identification of a thunderstorm as a source of short period gravity waves in the upper atmospheric nightglow emissions, Planet. Space Sci., 36, 975-985, 1988.
Taylor, M.J., and M.J. Hill, Near infrared imaging of hydroxyl wave structure over an ocean site at low latitudes, Geophys. Res. Lett., 18, 1333-1336, 1991.
Taylor, M.J., D.N. Turnbull and R.P. Lowe, Coincident imaging and spectrometric observations of zenith OH nightglow structure, Geophys. Res., Lett., 18, 1349-1352, 1991.
Taylor, M.J., V. Taylor and R. Edwards, "An Investigation of Thunderstorms as a Source of Short Period Mesospheric Gravity Waves," in The Upper Mesosphere and Lower Thermosphere: A Review of Experiment and Theory, AGU Geophysical Monograph 87, p 177, American Geophysical Union, Washington, D.C., 1995.
Yee, J.-H., R. Niciejewski and M.Z. Luo, Observations of ![]() and OH nightglow during the Aloha-90 campaign, Geophys. Res. Lett., 18, 1357-1360, 1991.
and OH nightglow during the Aloha-90 campaign, Geophys. Res. Lett., 18, 1357-1360, 1991.
Tables
Table 1. OH (X 2) Meinel Vibration-Rotation Bands
720 < < 1000 nm
|
Band |
, nm(Q-branch) |
Relative Intensity |
|
v=3 |
||
|
(3-0) |
966 (R-branch) |
2 |
|
v =4 |
||
|
(9-5) |
1000 |
3 |
|
(8-4) |
940 |
8 |
|
(7-3) |
883 |
7 |
|
(6-2) |
834 |
5 |
|
(5-1) |
792 |
6 |
|
(4-0) |
753 |
3 |
|
v =5 |
||
|
(9-4) |
776 |
10 |
|
(8-3) |
728 |
6 |
Source: Pearse and Gaydon, 1976.
Table 2. Comparison of Reported Gravity Wave Parameters
|
l h (km) |
l z (km) |
t (min) |
cph (m/s) |
cgh (m/s) |
cgz (m/s) |
D t (min) |
j (deg) |
|
|
Before 0430 |
50 |
30 |
10 |
85 |
58 |
27 |
43 |
31 |
|
After 0500 |
40 |
20 |
11 |
61 |
48 |
24 |
49 |
27 |
|
MSX 11/13 |
25 |
16 |
9 |
46 |
32 |
21 |
55 |
33 |
|
MSX 10/2 |
50 |
18 |
15 |
56 |
50 |
18 |
64 |
20 |
|
T&H 1988 |
26 |
7 |
17 |
38 |
23 |
11 |
105 |
15 |
l h: Horizontal wavelength
l z: Vertical wavelength
t : Wave period
cph: Wave horizontal phase speed
cgh: Wave horizontal group speed
cgz: Wave vertical group speed
D t: Wave-energy propagation time from 15 to 85 km altitude
j : Elevation angle of group-velocity vector
Figures
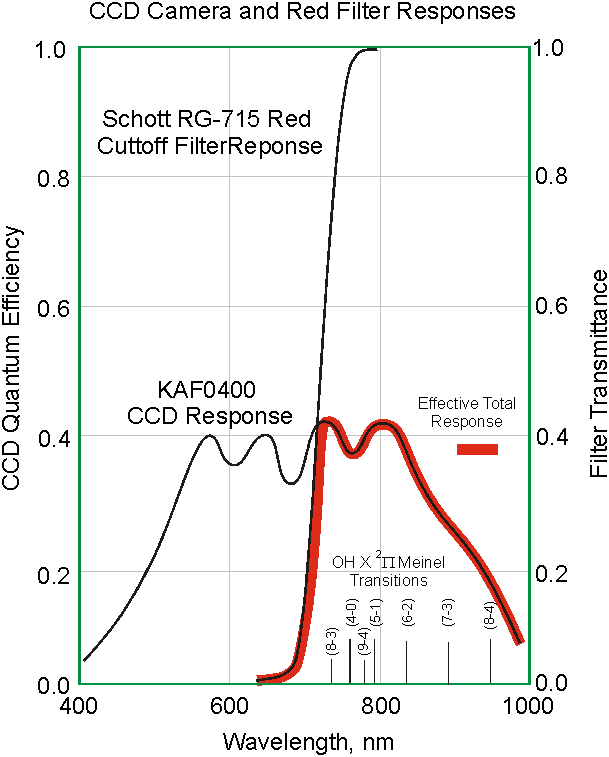
Figure 1. CCD and cutoff filter responses as functions of wavelength, and the combined response pass band. Also shown are locations of the band heads of the OH (X 2
) Dv=4,5 Meinel sequences within the combined response pass band.
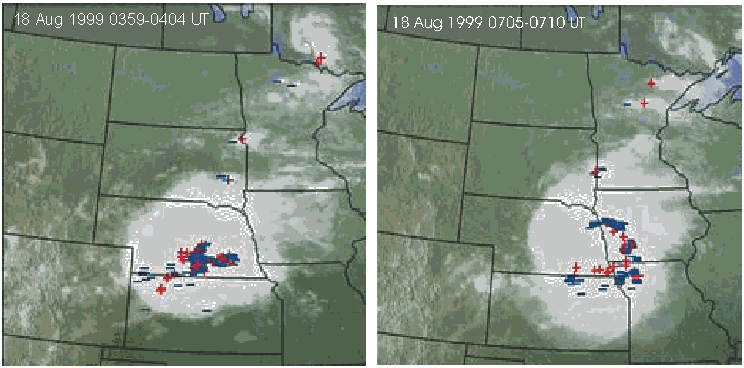
Figure 2. Thunderstorm over central Nebraska studied during the night of 18 August, 1999. The two maps show the same storm separated by three hours. Superimposed atop the maps are 5 min averages of lightning activity. Blue denotes negative CG lightning, and red denotes positive CG lightning. Adapted from near-real time maps downloaded from http://www.weather.com

Figure 3. Outwardly expanding concentric ripples observed in OH emissions using 25 sec time exposure in the NIR. The field of view is approximately 60H x 40V deg. The dashed lines labeled "analysis strip" delineate the region used to create Figure 5. The city lights of Custer, SD and lights from the Crazy Horse Monument are labeled at the bottom of the large plot. The stars in the figure are used to fix the viewing direction using the Smithsonian Star Catalog. The sprite on the main image is shown in the inset in more detail as it was recorded by a separate ICCD camera.

Figure 4. GOES weather image with image of Figure 3 mapped to a flat surface and laid over the storm. The outward expanding ripples are seen to be centered on the underlying thunderstorm. See text for description of the mapping projection used.

Figure 5. Color-coded position-time plot of emission intensity along the horizontal analysis strip indicated in Figure 2. The color axis runs from purple-red-yellow. The waves appear to emanate propagate outward and upward from the central vertical region of the figure. The wavelengths and periods of the waves are readily discernible from this plot, yielding a phase speed of about 60 km/sec. The right-to-left drift of the bright (yellow) central region with increasing time is interpreted to track the movement of the underlying thunderstorm across Nebraska. Stars and sprites that appear within the analysis strip are labeled.